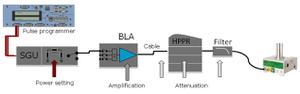
We can gain the pulse power at the pulse width if a pulse width has been calibrated at a given power level. This can determine the long pulse widths, shaped pulses, and off resonance pulses, others may be difficult to calibrate experimentally.
In electronics, the power level is represent by electronical gain expressed logarithmic decibel (dB) units. Historically the power level of nmr sequese pulse is defined by Power gain (dB). the equation between power gain and power is made as follows1:
$$
dB = 10log_10(\div{P_{out}}{P_{in}})
$$
and from Joule’s first law $P=V^2/R$ the above formula can be expressed by valtage as follows:
$$
dB = 20log_10(\div{V_{out}}{V_{in}})
$$
The field strength (pulse width) is antiproportional to voltage, therefore the dB relationship between pulse width and pulse power can be derived:
$$
dB_2 - dB_1 = 20log_10( \div{width1}{width2} )
$$
This formula assumes prefect linearity of the amplifiers and assumes the shape of pulse1 and pulse2. The non-linearity ratio can be calibrateb by two fold of 90 pulse width at dB - 6 and 90 pulse width at dB2.
The shaped pulses also can be calculated mathematically2.
Note: From Topspin 3.0 above, the Bruker use the -dBW, this use the 1W repond to 0 dBW, the formula is as follow:
$$
-dBW = -10 log_10(Watt/1W)
$$